. For what values of x is f'(x) = 0 ? Begin by differentiating the function using the product rule. Then
= 0 .
It follows that
or 4x+3 = 0 .
Thus, the values of x which solve f'(x) = 0 are
or 
.
Click HERE to return to the list of problems.
SOLUTION 14 : Consider the function 
. For what values of x is f'(x)=0 ? Begin by differentiating the function using the product rule. Then
= 0 .
It follows that
or -x+1 = 0 .
But 
can never be zero since an exponential is always positive.
Thus, the only values of x which solve f'(x) = 0 are
x = 0 or x = 1 .
Click HERE to return to the list of problems.
SOLUTION 15 : Consider the function 
. For what values of x is f'(x)=0 ? Begin by differentiating the function using the product rule. Then
= 0 .
It follows that
x = 0 or 
.
But x = 0 is not in the domain of function f since 
is not defined.
Thus, the only possibility is
iff
iff
iff
iff
.
Click HERE to return to the list of problems.
SOLUTION 16 : Prove that
.
Group functions f and g and apply the ordinary product rule twice. Then
= f'(x) g(x) h(x) + f(x) g'(x) h(x) + f(x) g(x) h'(x) .
Here is an easy way to remember the triple product rule. Each time differentiate a different function in the product. Then add the three new products together.
Click HERE to return to the list of problems.
SOLUTION 17 : Differentiate 
. Differentiate y using the triple product rule. Then
.
Click HERE to return to the list of problems.
SOLUTION 18 : Consider the function 
. For what values of x is f'(x)=0 ? Begin by differentiating f using the triple product rule. Then
(Now factor out the common terms of 
, and 
.)
.
It follows that
or 
.
But is never zero since exponentials are always positive. Thus, the values of x which solve f'(x)=0 are
or (using the quadratic formula)
.
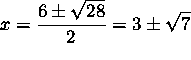Click HERE to return to the list of problems.
SOLUTION 19 : Find an equation of the line tangent to the graph of
at 
. If then
so that the tangent line passes through the point
. The slope of the tangent line follows from the derivative of y . Then
.
The slope of the line tangent to the graph at is
.
Thus, an equation of the tangent line is
or y=x .
Click HERE to return to the list of problems.
SOLUTION 20 : Find an equation of the line perpendicular to the graph of
at 
. If then
so that the tangent line
passes through the point 
. The slope of the line perpendicular to
the graph of f will be perpendicular to the line tangent to the graph of f . Thus, we need to first
compute the derivative of f . Then
.
Thus, the slope of the line tangent to the graph at is
.
Thus, the slope of the line perpendicular to the graph at is
and an equation of this line is
.
Click HERE to return to the list of problems.
SOLUTION 21 : Find all points (x, y) on the graph of 
with tangent lines parallel to the line y + x = 12 . The slope of the given line y = 12-x is y'=-1 . Thus, we need to find all values x in the domain of f which satisfy f'(x)=-1 . The derivative of f is
.
If 
then 
. It follows that
iff
iff
iff
iff
x(9x + 8) = 0 .
Thus,
x = 0 or 9x + 8 = 0
so that
x = 0 or 
.
But x=0 cannot be a solution since 
. Therefore, the only solution is the point
and
.
Click HERE to return to the list of problems.