Limits Using the Squeeze Principle

LIMITS OF FUNCTIONS USING THE SQUEEZE PRINCIPLE

The following problems involve the algebraic computation of limits using the Squeeze Principle, which is given below.

SQUEEZE PRINCIPLE : Assume that functions f , g , and h satisfy

and

Then

(NOTE : The quantity A may be a finite number, , or . The quantitiy L may be a finite number, , or .)

The Squeeze Principle is used on limit problems where the usual algebraic methods (factoring, conjugation, algebraic manipulation, etc.) are not effective. However, it requires that you be able to ``squeeze'' your problem in between two other ``simpler'' functions whose limits are easily computable and equal. The use of the Squeeze Principle requires accurate analysis, deft algebra skills, and careful use of inequalities.

PROBLEM 1 : Compute .
Click HERE to see a detailed solution to problem 1.
PROBLEM 2 : Compute .
Click HERE to see a detailed solution to problem 2.
PROBLEM 3 : Compute .
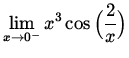Click HERE to see a detailed solution to problem 3.
PROBLEM 4 : Compute .
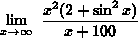Click HERE to see a detailed solution to problem 4.
PROBLEM 5 : Compute .
Click HERE to see a detailed solution to problem 5.
PROBLEM 6 : Compute .
Click HERE to see a detailed solution to problem 6.
PROBLEM 7 : Compute .
Click HERE to see a detailed solution to problem 7.
PROBLEM 8 : Assume that exists and . Find .
Click HERE to see a detailed solution to problem 8.
PROBLEM 9 : Consider a circle of radius 1 centered at the origin and an angle of radians, , in the given diagram.

a.) By considering the areas of right triangle OAD, sector OAC, and right triangle OBC, conclude that
.
b.) Use part a.) and the Squeeze Principle to show that

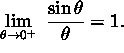
Click HERE to see a detailed solution to problem 9.
PROBLEM 10 : Assume that

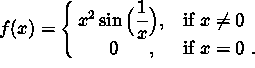
Show that f is continuous at x=0 .
Click HERE to see a detailed solution to problem 10.