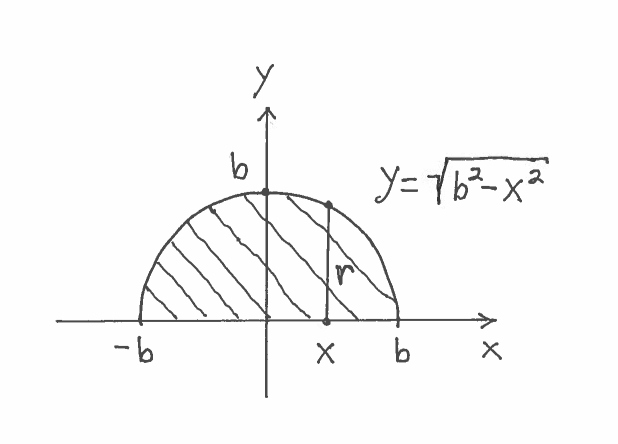
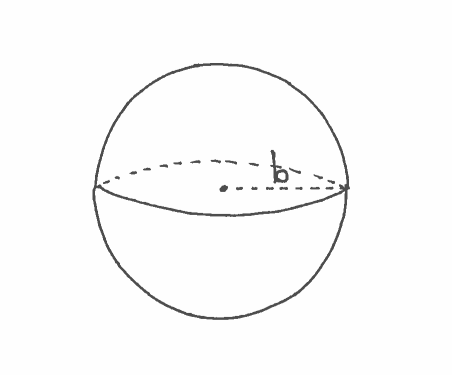
SOLUTION 5: Consider the region enclosed by the graph of the semi-circle $ y= \sqrt{ b^2 - x^2} $ on the interval $ [-b, b] $. Revolving this region about the $x$-axis will create the appropriate sphere of radius $b$. Here are a carefully labeled sketch of the region, a rough sketch of the resulting Solid of Revolution, and a circular cross-section at $x$ of radius $r$. It is IMPORTANT to mark BOTH $x$ and $r$ in the sketch of the region !!!
 $ \ \ \ $
$ \ \ \ $
 $ \ \ \ $
$ \ \ \ $
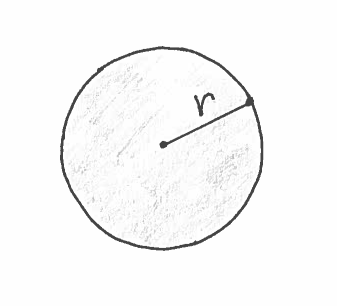
The area of the circular cross-section is
$$ A(x)= \pi r^2 = \pi (\sqrt{ b^2 - x^2} \ )^2 = \pi (b^2 - x^2) $$
Thus the total volume of this Solid of Revolution is
$$ Volume = \int_{-b}^{b} \pi (b^2 - x^2) \ dx $$
$$ = \int_{-b}^{b} ( \pi b^2 - \pi x^2) \ dx $$
$$ = \Big( \pi b^2 \cdot x - \pi \cdot {1 \over 3}x^3 \Big) \Big|_{-b}^{b} $$
$$ = \Big( \pi b^2 (b) - \pi {1 \over 3}(b)^3 \Big) - \Big( \pi b^2 (-b) - \pi {1 \over 3}(-b)^3 \Big) $$
$$ = {2 \over 3} \pi b^3 - ( - {2 \over 3} \pi b^3 ) $$
$$ = {4 \over 3} \pi b^3 $$
i.e.,
$$ Volume = {4 \over 3} \pi b^3 $$
Click HERE to return to the list of problems.
 $ \ \ \ $
$ \ \ \ $
 $ \ \ \ $
$ \ \ \ $

 $ \ \ \ $
$ \ \ \ $
 $ \ \ \ $
$ \ \ \ $
